What Are Triangles In Geometry? Types & Examples
Triangles, a shape we often see in our everyday lives, have numerous practical uses. In this article, let’s learn what triangles are and how they are classified based on their characteristics.
What is a triangle?
A triangle represents the simplest form of polygons. It is the most basic closed shape that can be formed using straight lines. Triangles also serve as fundamental building blocks for 3D objects like prisms, pyramids, and tetrahedrons, forming their faces. Each triangle consists of three interior angles, three sides, and three vertices.
Numerous rules mathematically capture the relationship between the various features of triangles. Some of them are:
- The sum of three interior angles in a triangle is 180 degrees.
- The sine rule captures the ratio between the angles of a triangle and their corresponding sides.
- The Cosine rule is another formula that links the lengths of a triangle’s sides to one of its angles.
- The well-known Pythagoras Theorem, a specific case of the Cosine Rule, applies exclusively to right-angled triangles.
- The sum of the lengths of two sides of a triangle must always exceed the length of the third side.
Triangles can be classified into several categories based on their angle sizes and other characteristics, which we will explore in the following section.
Triangles classified based on their angles
- Acute triangle: A triangle is classified as acute if all three of its interior angles are less than 90 degrees. Consider for example a triangle with angles 40,60, and 80, this is an acute triangle. Remember that as discussed above the sum of angles must always be 180, therefore a triangle with three 30-degree angles is impossible.
- Right Triangle: The right triangle is one of the most studied triangles in geometry. This type of triangle has one right angle – that is, an angle of 90 degrees – between two of its sides. The Pythagoras theorem describes the relationship between the length of the three sides of a right triangle. Additionally, trigonometric ratios, such as Sine, Cosine, And Tangent are defined for right triangles based on the ratios of sides and the angle.
- Obtuse Triangle:An obtuse triangle is defined by having one obtuse angle as one of its interior angles. As mentioned earlier, the sum of the interior angles of all triangles is 180 degrees hence a triangle can only have a single obtuse angle.

Triangles classified based on their sides
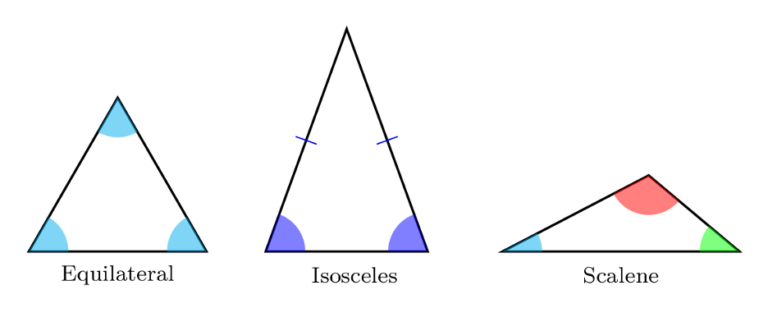
- Equilateral triangle: An equilateral triangle is the name given to a regular triangle. An equilateral triangle has sides of identical length. Each angle in an equilateral triangle measures 60 degrees, making it highly symmetrical. Equilateral triangles are frequently seen in everyday structures like 3-leg stools and tripods, where the equidistant legs form an equilateral triangle, contributing to the object’s stability.
- Isosceles Triangle: If only two of the three sides of a triangle are of equal length, then it is known as an isosceles triangle. The angle between these two equal sides is called the vertex angle. The two angles apart from the vertex angle are equal in size. Due to these features, the bisector of the vertex angle acts as the single line of symmetry in isosceles triangles.
- Scalene Triangle: A scalene triangle is characterized by having all sides of different lengths. Consequently, this means that the three interior angles of a scalene triangle are also distinct from each other.
Area of Triangles
The area of a triangle, similar to any two-dimensional shape, is the amount of space it occupies on a 2d plane. To determine the area of triangles there is a universal formula: ½ * base * perpendicular height.
This formula originates from the understanding that a triangle’s area is always half that of the smallest rectangle that can enclose it. In such a scenario, the smallest rectangle that surrounds the triangle shares two vertices with the triangle, as illustrated in the diagram below.
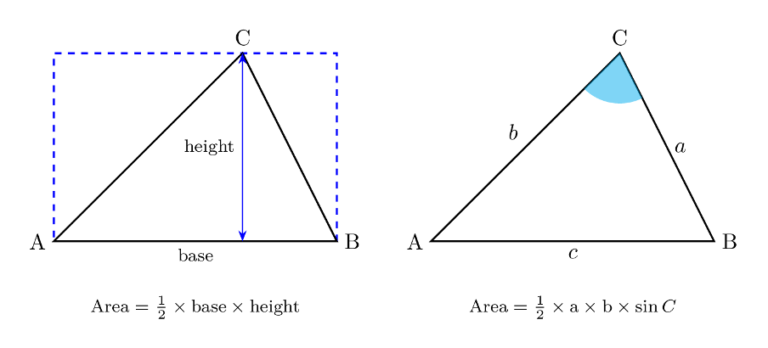
As shown above, the area of the triangle is half the area of the rectangle, i.e. ½ of length * width, which would be equivalent to the formula mentioned earlier. Another useful formula for finding a triangle’s area, particularly when you know the lengths of two sides and the included angle, is ½ × a × b × Sin(C).
Perimeter of Triangles
The perimeter of a shape is the total length of the boundary. In the case of a triangle, as with any polygon, the perimeter is determined by summing the lengths of all its sides. When some side lengths are not directly known various theorems can be used to deduce the lengths. These theorems include:
- Sine Rule
- Cosine Rule
- Pythagoras Theorem
Real-life applications of triangles
Extending beyond theoretical geometry the seemingly simple shape of a triangle finds extensive and crucial applications in various practical scenarios. Here are some key real-life applications of triangles:
- Triangulation – a method that leverages the properties of triangles, triangulation is used in surveying and navigation to determine distances and map out areas. This method is also integral to the functionality of GPS technology.
- Structural Stability: Triangles play a vital role in providing stability to load-bearing structures such as bridges, roofs, and cranes. Their inherent strength and rigidity make them essential components in architectural and engineering designs.

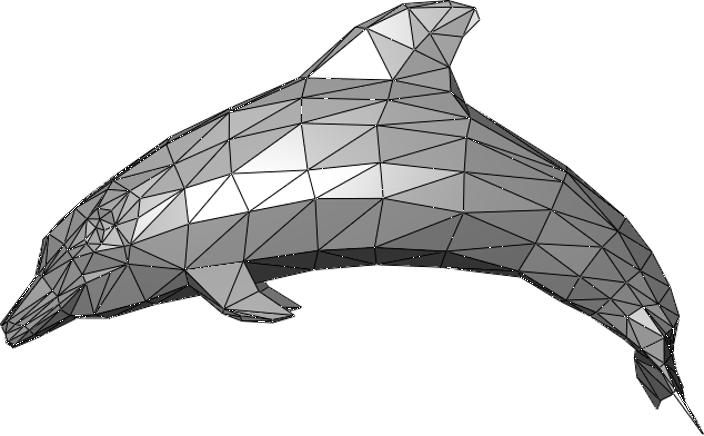
3. 3d-rendering – In 3d animation and games triangles serve as the building blocks of textures, terrains, 3d models, etc. Their simplicity makes it efficient for computers to generate triangles, thereby improving the efficiency of the rendering process and decreasing the time to create intricate objects.